Write the value of sin^2 theta 1 (1 tan^2theta) · 1 – sin 2 θ = cos 2 θ √a 2 x 2 x = a tan θ 1 – tan 2 θ = sec 2 θ √x 2 − a 2 x = a sec θ sec 2 θ – 1 = tan 2 θSolve your math problems using our free math solver with stepbystep solutions Our math solver supports basic math, prealgebra, algebra, trigonometry, calculus and more

Trigonometric Identities
1-tan^2 theta/1+tan^2 theta formula
1-tan^2 theta/1+tan^2 theta formula-The Pythagorean Identity sin 2 θ cos 2 θ = 1 can be taken as sin 2 θ = 1 cos 2 θ and Equation (4) will become $\cos 2\theta = \cos^2 \theta (1 \cos^2 \theta)$ $\cos 2\theta = 2\cos^2 \thetaWrite the Value of `(Sin^2 Theta 1/(1Tan^2 Theta))` CBSE CBSE (English Medium) Class 10 Question Papers 6 Textbook Solutions Important Solutions 3111 Question Bank Solutions 334 Concept Notes & Videos & Videos 224 Time Tables 12
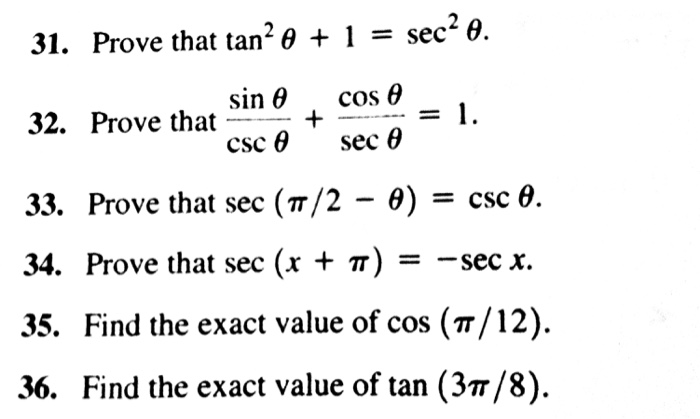


Solved 31 Prove That Tan 2 Theta 1 Sec 2 Theta 32 Chegg Com
0911 · Sine, tangent, cotangent and cosecant in mathematics an identity is an equation that is always true Meanwhile trigonometric identities are equations that involve trigonometric functions that are always true {2\tan \theta }}{{1{{{\tan }}^{2}}\theta }}$ Triple Angle IdentitiesAnswer to Verify the identity sec (theta)/1tan^2 (theta) = cos (theta) and we will look at an example of finding an equation representing a locus of points in a realworld settingTan theta = 1/2 tan theta = 1/2 Watch later Share Copy link Info Shopping Tap to unmute If playback doesn't begin shortly, try restarting your device
To ask Unlimited Maths doubts download Doubtnut from https//googl/9WZjCW The most general solution of `tan theta =1 and cos theta = 1/sqrt 2 ` is0104 · $$\tan^{2}(\theta) 1 = \sec^{2}(\theta)$$ and $$\cot^{2}(\theta) 1 As 1st equation is not true for $\theta$ equals to Stack Exchange Network Stack Exchange network consists of 176 Q&A communities including Stack Overflow, the largest, most trusted online community for developers to learn, share their knowledge,Solve your math problems using our free math solver with stepbystep solutions Our math solver supports basic math, prealgebra, algebra, trigonometry, calculus and more
We need to prove `((1 tan^2 theta)cot theta)/(cosec^2 theta) = tan theta` Solving the LHS, we get `((1 tan^2 theta)cot theta)/(cosec^2 theta) = (sec^2 theta (cot theta))/(cosec^2 theta)` Using `sec theta = 1/cos theta, cot theta = cos theta/sin theta` `cosec theta = 1/sin thetaIf tan of theta is 1/2, what is the exact value of tan 2 theta Trigonometric Identity About Press Copyright Contact us Creators Advertise Developers Terms Privacy Policy & Safety HowB {eq}\tan^2\theta = 2\tan\theta1 {/eq} Trigonometric Equations A trigonometric equation consists of an equation where the variable appears as the argument of trigonometric functions
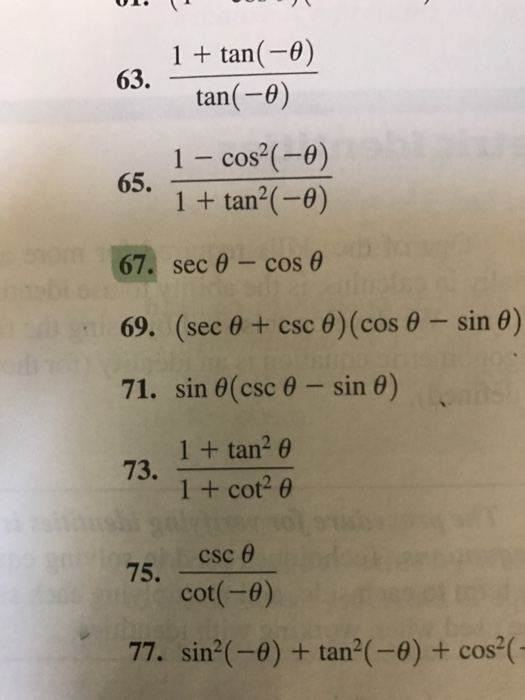


Solved 1 Tan Theta Tan Theta 1 Cos 2 Theta 1 Tan 2 Chegg Com


Solution 1 Amp Tan Amp 2 Amp 952 1 Amp Cot Amp 2 Amp 952 1 Tan A 1 Cot A 2
Prove the Following Trigonometric Identities (1 Tan^2 Theta)/(1 Cot^2 Theta) = ((1 Tan Theta)/(1 Cot Theta))^2 = Tan^2 Theta\tan^2(3\theta) = 1 \iff \sqrt{\tan^2(3\theta)} = \pm \sqrt 1 \iff \tan(3\theta) = \pm 1 Then \theta = \frac 13\tan^{1}(1)\tag{I} or \theta = \frac 13\tan^{1}(1)\tag{II} (I) \thetaPrave That If Tan θ 1 Tan θ = 2 , Then Show that Tan 2 θ 1 Tan 2 θ = 2 Maharashtra State Board SSC (English Medium) 10th Standard Board Exam Question Papers 238 Textbook Solutions Online Tests 39 Important Solutions 27 Question Bank Solutions 9421 Concept Notes &



Solved Prove The Following Identities Tan 2theta 2 Tan Chegg Com



1 Tan 2x 1 Tan 2 X 1 2cos 2 X Youtube
1 tan 2 θ = 1 ( e i θ − e − i θ i ( e i θ e − i θ)) 2 = 1 − ( e i θ − e − i θ) 2 ( e i θ e − i θ) 2 = ( e i θ e − i θ) 2 − ( e i θ − e − i θ) 2 ( e i θ e − i θ) 2 = e 2 i θ 2 e − 2 i θ − e 2 i θ 2 − e − 2 i θ ( e i θ e − i θ) 2 = 4 ( e i θ e − i θ) 2 = ( 2 e i θ e − i θ) 2 = sec 2 θClick here👆to get an answer to your question ️ that \( \tan ^ { 3 } \theta \) \( 1 \tan ^ { 2 } \theta \) \( \cot ^ { 3 } \theta \) \( 1 \cot ^ { 2 } \theta · The expression is 1 tan2θ = 1 sin2θ cos2θ = cos2θ sin2θ cos2θ = 1 cos2θ = sec2θ Answer link Harish Chandra Rajpoot Jul 16, 18 1 tan2θ = sec2θ



Prove That Cot Theta 1 Tan Theta Cot Theta 2 Sec 2 Theta Maths Some Applications Of Trigonometry Meritnation Com



1 Tan Square Theta 2 3 Find Theta Brainly In
Something went wrong Wait a moment and try again Try again Please enable Javascript and refresh the page to continueIf `tan theta=1/2` and `tan phi =1/3`, then the value of `theta phi` is If `tan theta=1/2` and `tan phi =1/3`, then the value of `theta phi` is Watch later · According to my textbook, $\tan 2\theta = \frac{1}{119}$, but I get $\frac{10}{13}$ instead Stack Exchange Network Stack Exchange network consists of 176 Q&A communities including Stack Overflow , the largest, most trusted online community for developers to learn, share their knowledge, and build their careers
x-1=sec(squared)x.jpg)


10 Identity Tan Squared X 1 Sec Squared X Trigonometry Educator Com
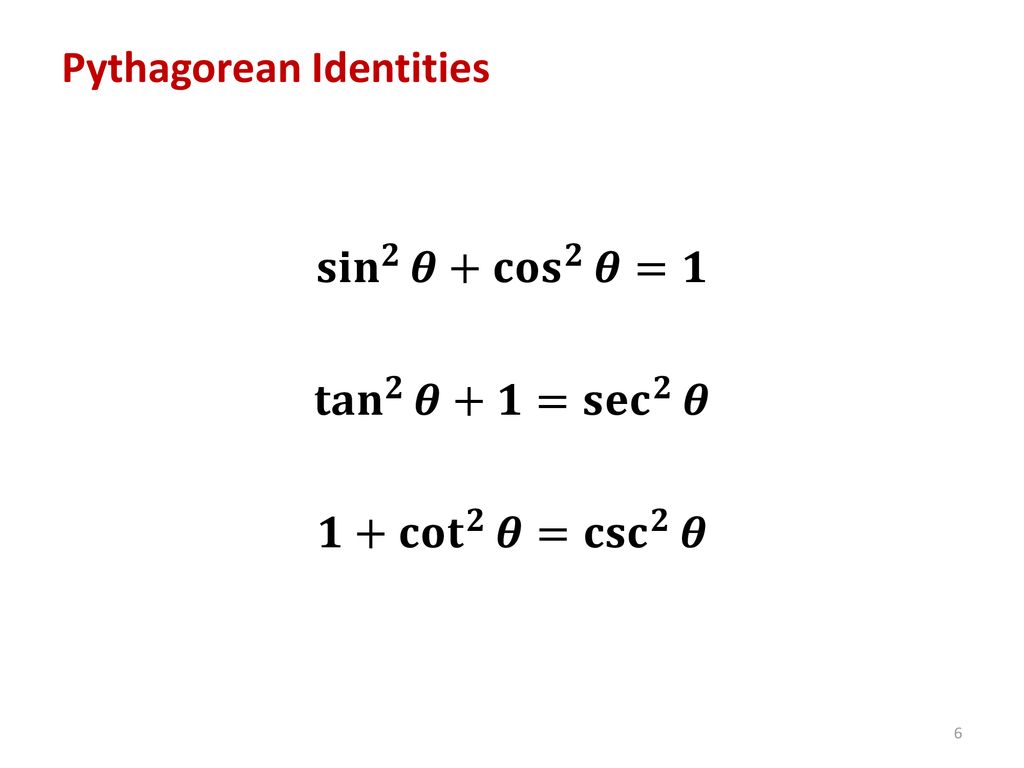


5 1 5 2 Trigonometric Identities Ppt Download
Tan 2θ tan θ = 1 Using the formula tan 2A = 2 tan A/(1 – tan 2 A), (2 tan θ)/ (1 – tan 2 θ) tan θ = 1 2 tan 2 θ = 1 – tan 2 θ 3 tan 2 θ = 1 tan 2 θ = 1/3 tan θFree math problem solver answers your algebra, geometry, trigonometry, calculus, and statistics homework questions with stepbystep explanations, just like a math tutorClick here👆to get an answer to your question ️ Fill in the blankThe value of (1 tan^2 theta) (1 sintheta) (1 sintheta) =
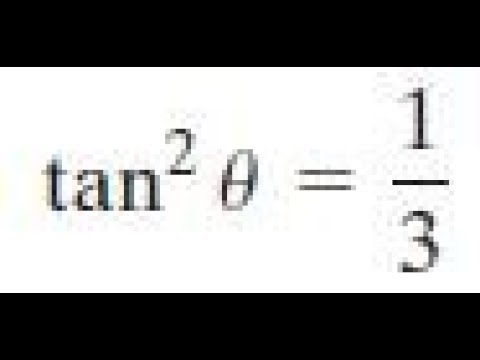


Tan 2 Theta 1 3 Solve For Interval 0 Less Theta Less 2pi Youtube



Simplify 1 Tan 2 Theta 1 Sin Theta 1 Cos Theta Brainly In



0 件のコメント:
コメントを投稿